"History is a vast early warning system." — Norman Cousins
The calendar has come to us from Ancient Egypt and we still use it to this day. It is a calendar based on Sothis (the star Sirius). The Egyptian pharaohs had to swear never to change the calendar! This Ancient calendar is, essentially, the most reasonable calendar ever devised in all human history. Its mathematical algorithm is determined by two cycles:
Both of these cycles were well known in the ancient times.
One description of the 4-year cycle that we know was found in the Canopus Decree.
The
Canopus Decree (stone plate with trilingual inscription,
"...on account of the procession of the rising of the Divine Sothis1 by one day in the course of 4 years,... it shall be that a year of 360 days and 5 days added to their end, so one day as Feast of the Benevolent Gods2 be from this day after every 4 years added to the 5 epagomenae before the new year..."
1 Divine Sothis: Sirius. 2 Benevolent Gods: The ruler Ptolemy III Euergetes and his wife Berenike.
This rule was also introduced in the Seleucid calendar adopted in 312 BC.
The origin of a 7-day cycle also dates back to antiquity. For example, a division of the year into fifty-two weeks of seven days each is clearly stated in the text of the Book of Jubilees. It says that when the Deluge ended, Noah was given "heavenly tablets", ordaining that:
"All the days of the commandment will be two and fifty weeks of days which will make the year complete. Thus it is engraven and ordained on the heavenly tablets..."
The Christian, Hebrew, and Islamic calendars all have a 7-day week. There is no record of the 7-day week cycle ever having been broken. Calendar changes and reform have never interrupted the 7-day cycles.
Two main versions of this Ancient calendar have existed in recent times: the Julian calendar and the Gregorian calendar. When the Roman general Gaius Julius Caesar came to Egypt in 48 BC, he was so impressed by the Egyptian calendar that he decided to introduce it into Roman Empire. He made his famous calendar reform in 45 BC. After that, the Ancient calendar has become to be known as the Julian calendar. The calendar Julius Caesar adopted was identical to the Alexandrian Aristarchus calendar of 239 BC. It consisted of a solar year of twelve months and of 365 days with an extra day every fourth year. Hence, the length of a year was approximated as 365.25 days.
(3 × 365 + 366) : 4 = 365.25
This gives an error of 1 day in approximately 128 years. The Julian calendar served western Europe for more than 1,500 years. For example, the Orthodox Church in Russia still uses it.
The calendar reform of Pope Gregory XIII (1582 AD) has added one additional rule to the Ancient calendar to increase its accuracy. In the Julian calendar, the rule was used: Gregorian reform added: Thus, the years 1600 and 2000 are leap years, but 1700, 1800, 1900, and 2100 are not. However, in the Julian calendar these years will be leap years. In each such case, the difference between Julian and Gregorian dates is increased by one day. Thus, the "Gregorian" calendar is not really "new"; it is only the Gregorian amendment to the Ancient calendar. I shall not use this amendment for decoding the Message (see Part 2).
If you want to compare dates of the Julian and Gregorian calendars, it is really quite simple. For conversion of a date of the Gregorian calendar to a date of the Julian calendar, we need to subtract 13 days (when converting the Gregorian dates from March 14, 1900 till March 14, 2100). Conversely, for conversion of a date of the Julian calendar to a date of the Gregorian calendar we need to add 13 days. For example, March 1, 2000 on the Julian calendar corresponds to (1+13=14) March 14, 2000 on the Gregorian calendar and vice versa.
Our calendar has years of 365 or 366 days. The year and the day are natural divisions of time. In parallel with this system, the concept of "weeks" groups the days in sets of 7. How can this be explained? The 7-day week is not a natural division of time. Look at a recent calendar, the year 2000.
The year 2000 is a leap year with a leap day, February 29.
Rule 1: The leap year has 366 days or 52 weeks and 2 days
366 = 7 × 52 + 2
The first day of year 2000 is Saturday and the last day is Sunday (that is, the next day of week. This rule is applicable for any leap year).
Rule 2: An ordinary (non-leap) year has 365 days or 52 weeks and 1 day
365 = 7 × 52 + 1
For example, 2003 is an ordinary year. It begins on Wednesday, and finishes on Wednesday. Any ordinary year begins and finishes at the same day of week.
Rule 3: All sequence of years are divided into four-year groups: 3 ordinary years and 1 leap year; 3 ordinary years and 1 leap year; etc.
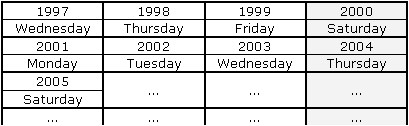
Using rules 1, 2, and 3, it is possible to write the table of all years thus:
Table 1
Here for each year, the day of week for the first day of a year (for January 1) is indicated. All leap years are located in the fourth column of Table 1. This is the table for the Gregorian calendar of January style (January 1 is a first day of a new year). Does this sequence of years contain a set of calendars that is constantly repeated? Yes, in this sequence of years there is a calendar cycle.
Julian Calendar of the March Style
Further, I shall use the Julian calendar of March style (March 1 is a first day of a new year). In the March style, an extra day — February 29 — is added (or not added) at the end of year (it is the last day of a year). Now it is possible to rewrite Table 1 for the Julian calendar of the March style so:
Table 2
Thereafter, all sequence is repeated. Days of the week for all dates of years 2025 and 1997 will be identical. That is, the calendar for 2025 will coincide with the calendar for 1997; the calendar for 2026 will coincide with the calendar for 1998 and so on. In the Julian calendar, the relationship between the days of the week and the dates of the year is repeated in cycles of 28 years. This period of 28 years is called a Solar cycle.
Shown in each cell of Table 2 are:
All leap years are located in the third column of Table 2. It does not change the average length of a year:
(365 + 365 + 366 + 365) : 4 = 365.25
All designs of "Perpetual" calendars use Table 2. Now I shall show how to use Table 2 to solve the calendar problems.
The Calendar Calculations
Throughout this article the operator "mod" will be used to signify the modulo or remainder operator. For example:
17 mod 7 = 3
This is because 17 divided by 7 equals 2 with a remainder of 3.
The Solar number of any year AD in a Solar cycle can be calculated thus:
(Y - 8) mod 28 = S
where: Y - year; S - Solar number of a year in a Solar cycle. If S = 0, it is 28-th year of a Solar cycle.
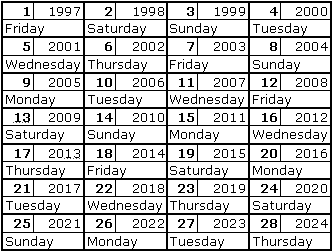
Using Table 2 we can determine a week day for March 1 of any year. For example, S for 2003 equals
(2003 - 8) mod 28 = 7
S = 7 corresponds to Friday (see Table 2). Therefore, March 1, 2003 of the Julian calendar was Friday. March 1, 2003 of the Julian calendar corresponds to March 14, 2003 of the Gregorian calendar. Therefore, March 14, 2003 of the Gregorian calendar was Friday. Check it out!
One more example. S for 2025 equals
(2025 - 8) mod 28 = 1
S = 1 corresponds to Friday (see Table 2). Friday, March 1, 2025 of the Julian calendar corresponds to Friday, on March 14, 2025 of the Gregorian calendar.
All analyses of the calendar structure usually end here, but it is only the beginning of my research.
The Calendar Message
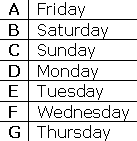
I began to study the matrix of the Solar cycle (Table 2). Now, the each day of week I shall designate by a letter:
Table 3
It is possible to rewrite Table 2 using these letters:
Table 4
Here, only the week days are used. The structure of Table 4 determines the algorithm of a Perpetual Calendar. It is the algorithm of the ancient calendar that has come to us from Ancient Egypt.
To be continued (see Part Two)
Copyright © 2000 Vladimir Pakhomov All
rights reserved. Except for the quotation of short passages for the purposes of
Updated: October 12, 2019
|